Sine waves.....

The Topics | Home

261

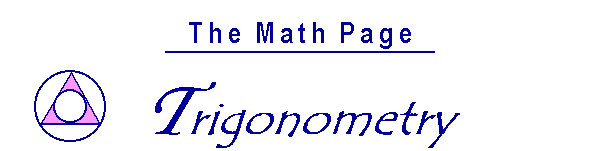
4

THE 30°-60°-90° TRIANGLE

THERE ARE TWO special triangles in trigonometry. One is the 30°-60°-90° triangle. The other is the isosceles right triangle. They are special because, with simple geometry, we can know the ratios of their sides. 

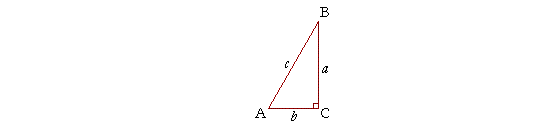
Theorem.  In a 30°-60°-90° triangle the sides are in the ratio 1 : 2 : .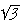

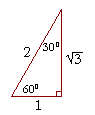
We will prove that below.

(For the definition of measuring angles by "degrees," see Topic 12.)

Note that the smallest side, 1, is opposite the smallest angle, 30°; while the largest side, 2, is opposite the largest angle, 90°.  (Theorem 6). (For, 2 is larger than .  Also, while 1 : : 2 correctly corresponds to the sides opposite 30°-60°-90°, many find the sequence 1 : 2 :  easier to remember.)

The cited theorems are from the Appendix, Some theorems of plane geometry.

Here are examples of how we take advantage of knowing those ratios.  First, we can evaluate the functions of 60° and 30°.

Example 1.   Evaluate cos 60°.

Answer.  For any problem involving a 30°-60°-90° triangle, the student should not use a table.  The student should sketch the triangle and place the ratio numbers.

Since the cosine is the ratio of the adjacent side to the hypotenuse, you can see that cos 60° = ½.

Example 2.   Evaluate sin 30°.

Answer.   According to the property of cofunctions (Topic 3), sin 30° is equal to cos 60°.   sin 30° = ½.

You can see that directly in the figure above.

Problem 1.   Evaluate sin 60° and tan 60°.

To see the answer, pass your mouse over the colored area.
To cover the answer again, click "Refresh" ("Reload").

The sine is the ratio of the opposite side to the hypotenuse. 

sin 60° =

2

= ½.

Lesson 5 of Algebra.

The tangent is ratio of the opposite side to the adjacent.

tan 60° =

1

= .

Problem 2.   Evaluate cot 30° and cos 30°.

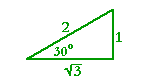
The cotangent is the ratio of the adjacent side to the opposite. 

Therefore, on inspecting the figure above, cot 30° =

1

= .

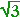Or, more simply, cot 30° = tan 60°.  Problem 1.

As for the cosine, it is the ratio of the adjacent side to the hypotenuse. Therefore,

cos 30° =

2

= ½.

Before we come to the next Example, here is how we relate the sides and angles of a triangle:

If an angle is labeled capital A, then the side opposite will be labeled small a.  Similarly for angle B and side b, angle C and side c.

Example 3.   Solve the right triangle ABC if angle A is 60°, and side c is 10 cm. 

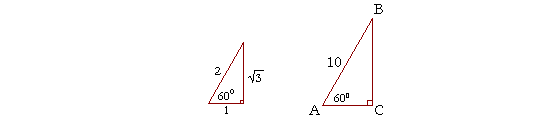
Solution.  To solve a triangle means to know all three sides and all three angles.  Since this is a right triangle, and angle A is 60°, then the remaining angle B is its complement, 30°.

Now in every 30°-60°-90° triangle, the sides are in the ratio 1 : 2 : , as shown on the right. Whenever we know the ratios of the sides, we can solve the triangle by the method of similar figures. 

And so in triangle ABC, the side corresponding to 2 has been multiplied by 5.  Therefore every side will be multiplied by 5.  Side bwill be 5 × 1, or simply 5 cm, and side a will be 5cm.

Alternatively, we could say that the side adjacent to 60° is always half of the hypotenuse.  Therefore, side b will be 5 cm.  Now, side b is the side that corresponds to 1.  And it has been multiplied by 5.  Therefore, side a must also be multiplied by 5.  It will be 5cm.

Whenever we know the ratio numbers, the student should use this method of similar figures to solve the triangle, and not the trigonometric Table.

(In Topic 6, we will solve right triangles the ratios of whose sides we do not know.)

Problem 3.   In the right triangle DFE, angle D is 30°, and side DF is 3 inches.  How long are sides d and f ?

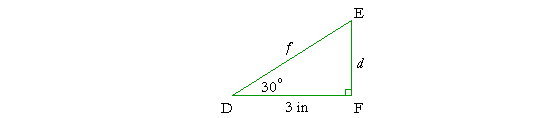
   The student should draw a similar triangle in the same orientation. Then see that the side corresponding to 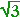 was multiplied by 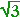. Therefore, each side will be multiplied by 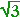. Side d will be 1 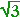= 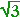. Side f will be 2.

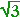Problem 4.   In the right triangle PQR, angle P is 30°, and side r is 1 cm.  How long are sides p and q ?

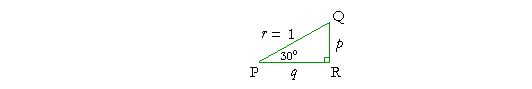
The side corresponding to 2 has been divided by 2.   Therefore, each side must be divided by 2.  Side p will be ½, and side q will be ½.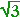

Problem 5.   Solve the right triangle ABC if angle A is 60°, and the hypotenuse is 18.6 cm.