One and one make two.......

The "Unit Circle" is a circle with a radius of 1.

Being so simple, it is a great way to learn and talk about lengths and angles.
The center is put on a graph where the x axis and y axis cross, so we get this neat arrangement here.

Sine, Cosine and Tangent

Because the radius is 1, we can directly measure sine, cosine and tangent.

What happens when the angle, θ, is 0°?

cos 0° = 1, sin 0° = 0 and tan 0° = 0

What happens when θ is 90°?

cos 90° = 0, sin 90° = 1 and tan 90° is undefined

Try It Yourself!

Have a try! Move the mouse around to see how different angles (in radians or degrees) affect sine, cosine and tangent

The "sides" can be positive or negative according to the rules of Cartesian coordinates. This makes the sine, cosine and tangent change between positive and negative values also.

Also try the Interactive Unit Circle.

Pythagoras

Pythagoras' Theorem says that for a right angled triangle, the square of the long side equals the sum of the squares of the other two sides:

x² + y² = 1²

But 1² is just 1, so:

x² + y² = 1
(the equation of the unit circle)

Also, since x=cos and y=sin, we get:

(cos(θ))² + (sin(θ))² = 1

a useful "identity"

Important Angles: 30°, 45° and 60°

You should try to remember sin, cos and tan for the angles 30°, 45° and 60°.
Yes, yes, it is a pain to have to remember things, but it will make life easier when you know them, not just in exams, but other times when you need to do quick estimates, etc.

These are the values you should remember!

Angle	Sin	Cos	Tan=Sin/Cos
30°			1 √3 = √3 3
45°			1
60°			√3

How To Remember?

To help you remember, think "1,2,3" :

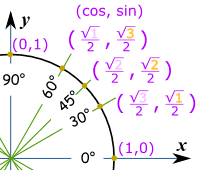
	sin(30°)  =	√1	=	1	(because √1 = 1)
		2		2

	sin(45°)  =	√2
		2

	sin(60°)  =	√3
		2

And cos goes "3,2,1"

	cos(30°)  =	√3
		2

	cos(45°)  =	√2
		2

	cos(60°)  =	√1	=	1	(because √1 = 1)
		2		2

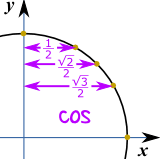
Just 3 Numbers

In fact, knowing 3 numbers is enough:	1	,	√2	and	√3
	2		2		2

Because they work for cos as well as sin:

What about tan?

Well, tan = sin/cos so we can calculate it like this:

tan(30°) = sin(30°)  =  1/2  =  1 cos(30°) √3/2 √3		But writing 1/√3 may cost you marks (see Rational Denominators), so instead use √3/3
tan(45°) = sin(45°)  =  √2/2  = 1  cos(45°) √2/2
tan(60°) = sin(60°)  =  √3/2  = √3  cos(60°) 1/2