What does a triangle with one side of i...................and another of a real number..........the square root of 2.......look like??  The complex plane...........

Either Part Can Be Zero

So, a Complex Number has a real part and an imaginary part.
But either part can be 0, so all Real Numbers and Imaginary Numbers are also Complex Numbers.

Complex Number	Real Part	Imaginary Part
3 + 2i	3	2
5	5	0
−6i	0	−6

Complicated?

Complex does not mean complicated.
It means the two types of numbers, real and imaginary, together form a complex, just like a building complex (buildings joined together).

A Visual Explanation

You know how the number line goes left-right?
Well let's have the imaginary numbers go up-down:

And we get the Complex Plane

And a complex number can now be shown as a point:

The complex number 3 + 4i

Adding

To add two complex numbers we add each part separately:

(a+bi) + (c+di) = (a+c) + (b+d)i

Example: add the complex numbers 3 + 2i and 1 + 7i

• add the real numbers, and
• add the imaginary numbers:

(3 + 2i) + (1 + 7i)
= 3 + 1 + (2 + 3)i
= (4 + 9i)

Let's try one visually:

Example: add the complex numbers 3 + 5i and 4 − 3i

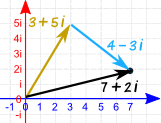
(3 + 5i) + (4 − 3i)
= 3 + 4 + (5 − 3)i
= 7 + 2i

Multiplying

To multiply complex numbers:

Each part of the first complex number gets multiplied by
each part of the second complex number

Just use "FOIL", which stands for "Firsts, Outers, Inners, Lasts" (see Binomial Multiplication for more details):

	Firsts: a × c Outers: a × di Inners: bi × c Lasts: bi × di
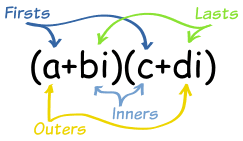(a+bi)(c+di) = ac + adi + bci + bdi2