9/8 would be the 1st 1/8 greater than pi..........pi is just 8/8...........8 + 8 = 16.....fractal patterns galore........9/8 is imp. why? 9 * 8 = 72..........the rule of 72 in finance.......72/2 = 36.............36 years for your money to double with a 2% interest rate..........72/3 = 24..........11 + 13 = 24......17 + 19 = 36............the 1st 4 primes in double digits.....11, 13, 17, 19.....all four sum to 60...........36....a truncated full circle.............end to beg and the two middle sum to 2 30s......like 2, 3...............72/4 = 18..........18 again...............like 1/8.....like 1/8 = 0.125.........1 + 2 + 5 = 8.......symmetry..........pi = 3.14...............3 + 1 + 4 = 8........8......the most stable electron orbital...............8 electrons is the most stable to be in a given distance from the nucleus..........also imp. b/c 3.141..............3.14......makes sense............b/c 1 repeats.......................18 is the 3rd integer from successive divisors of interest rates..1 is the 1st number....but if u divide 72 by 1 it is just 72.............
3.3. Harmonic Series I: Timbre and Octaves*
Have you ever wondered how a trumpet plays so many different notes with only three valves, or how a bugle plays different notes with no valves at all? Have you ever wondered why an oboe and a flute sound so different, even when they're playing the same note? What is a string player doing when she plays "harmonics"? Why do some notes sound good together while other notes seem to clash with each other? The answers to all of these questions have to do with the harmonic series.
Most musical notes are sounds that have a particular pitch. The pitch depends on the main frequency of the sound; the higher the frequency, and shorter the wavelength, of the sound waves, the higher the pitch is. But musical sounds don't have just one frequency. Sounds that have only one frequency are not very interesting or pretty. They have no more musical color than the beeping of a watch alarm. On the other hand, sounds that have too many frequencies, like the sound of glass breaking or of ocean waves crashing on a beach, may be interesting and even pleasant. But they don't have a particular pitch, so they usually aren't considered musical notes.
Figure 3.13. Frequency and Pitch
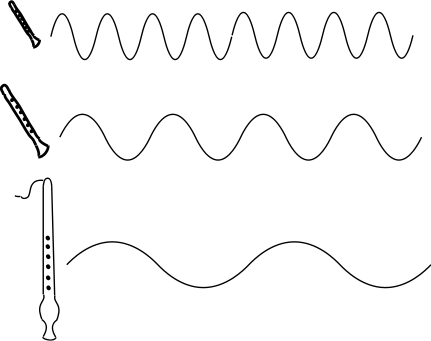
The shorter the wavelength, and higher the frequency, the higher the note sounds.
When someone plays or sings a musical tone, only a very particular set of frequencies is heard. Each note that comes out of the instrument is actually a smooth mixture of many different pitches. These different pitches are called harmonics, and they are blended together so well that you do not hear them as separate notes at all. Instead, the harmonics give the note its color.
What is the color of a sound? Say an oboe plays a middle C. Then a flute plays the same note at the same dynamic level as the oboe. It is still easy to tell the two notes apart, because an oboe sounds different from a flute. This difference in the sounds is the color, or timbre (pronounced "TAM-ber") of the notes. Like a color you see, the color of a sound can be bright and bold or deep and rich. It can be heavy, light, dark, thin, smooth, murky, or clear. Some other words that musicians use to describe the timbre of a sound are: reedy, brassy, piercing, mellow, hollow, focussed, transparent, breathy (pronounced BRETH-ee) or full. Listen to recordings of a violin and a viola. Although these instruments are quite similar, the viola has a noticeably "deeper" and the violin a noticeably "brighter" sound that is not simply a matter of the violin playing higher notes. Now listen to the same phrase played by an electric guitar, an acoustic guitar with twelve steel strings and an acoustic guitar with six nylon strings. The words musicians use to describe timbre are somewhat subjective, but most musicians would agree with the statement that, compared with each other, the first sound is mellow, the second bright, and the third rich.
No comments:
Post a Comment