Middle terms..........like the Bell curve...........m = 0...................dead center..................half way through...................like 1/2..............34.1 on either side........of the highest point of the Bell curve.............half way through.........68.2%...............like the fundamental.......2 and 68........like half the square root of 3.......0.86.............8 + 6 = 14................6 + 8 = 14................like 1.414.........from the square root of 2.........................68.2..............man oh man..........two garbled pi's.........like 2pi........a 2 and a 3............like 2, 3..........the start of all primes..............and the only time ever two primes are a digit apart........

2..........as a fundamental.................2, 3, 5.................taken apart.................2 then.......3, 5.............1 then 2........like 1/2..........the critical line.........

Proof of the Euler product formula for the Riemann zeta function

From Wikipedia, the free encyclopedia

Jump to: navigation, search

Leonhard Euler proved the Euler product formula for the Riemann zeta function in his thesis Variae observationes circa series infinitas (Various Observations about Infinite Series), published by St Petersburg Academy in 1737.^[1][2]

Contents

 [hide] 

• 1 The Euler product formula
• 2 Proof of the Euler product formula
• 3 The case ${\displaystyle s=1}$
• 4 Another proof
• 5 References
• 6 Notes

The Euler product formula[edit]

The Euler product formula for the Riemann zeta function reads

${\displaystyle \zeta (s)=\sum _{n=1}^{\infty }{\frac {1}{n^{s}}}=\prod _{p{\text{ prime}}}{\frac {1}{1-p^{-s}}}}$

where the left hand side equals the Riemann zeta function:

${\displaystyle \zeta (s)=\sum _{n=1}^{\infty }{\frac {1}{n^{s}}}=1+{\frac {1}{2^{s}}}+{\frac {1}{3^{s}}}+{\frac {1}{4^{s}}}+{\frac {1}{5^{s}}}+\ldots }$

and the product on the right hand side extends over all prime numbers p:

${\displaystyle \prod _{p{\text{ prime}}}{\frac {1}{1-p^{-s}}}={\frac {1}{1-2^{-s}}}\cdot {\frac {1}{1-3^{-s}}}\cdot {\frac {1}{1-5^{-s}}}\cdot {\frac {1}{1-7^{-s}}}\cdots {\frac {1}{1-p^{-s}}}\cdots }$

Proof of the Euler product formula[edit]

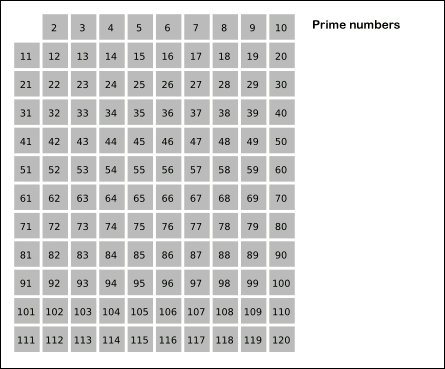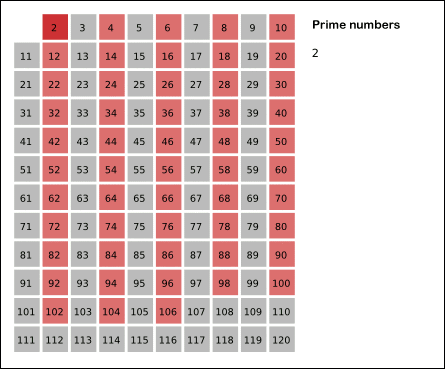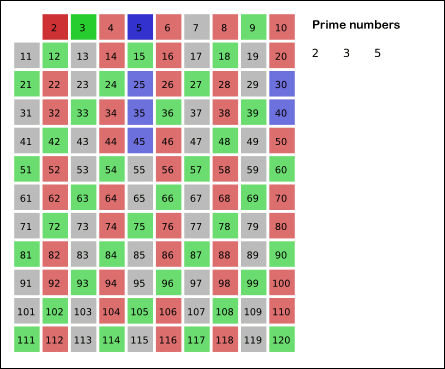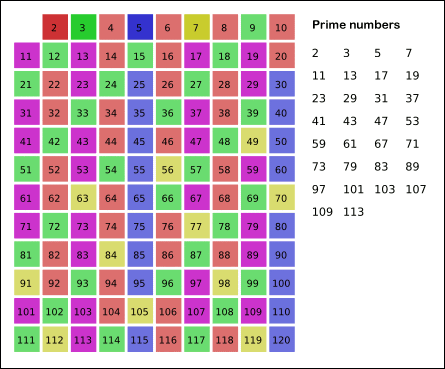
The method of Eratosthenes used to sieve out prime numbers is employed in this proof.

This sketch of a proof only makes use of simple algebra. This was originally the method by which Euler discovered the formula. There is a certain sieving property that we can use to our advantage:

${\displaystyle \zeta (s)=1+{\frac {1}{2^{s}}}+{\frac {1}{3^{s}}}+{\frac {1}{4^{s}}}+{\frac {1}{5^{s}}}+\ldots }$

${\displaystyle {\frac {1}{2^{s}}}\zeta (s)={\frac {1}{2^{s}}}+{\frac {1}{4^{s}}}+{\frac {1}{6^{s}}}+{\frac {1}{8^{s}}}+{\frac {1}{10^{s}}}+\ldots }$

Subtracting the second equation from the first we remove all elements that have a factor of 2:

${\displaystyle \left(1-{\frac {1}{2^{s}}}\right)\zeta (s)=1+{\frac {1}{3^{s}}}+{\frac {1}{5^{s}}}+{\frac {1}{7^{s}}}+{\frac {1}{9^{s}}}+{\frac {1}{11^{s}}}+{\frac {1}{13^{s}}}+\ldots }$

Repeating for the next term:

${\displaystyle {\frac {1}{3^{s}}}\left(1-{\frac {1}{2^{s}}}\right)\zeta (s)={\frac {1}{3^{s}}}+{\frac {1}{9^{s}}}+{\frac {1}{15^{s}}}+{\frac {1}{21^{s}}}+{\frac {1}{27^{s}}}+{\frac {1}{33^{s}}}+\ldots }$

Subtracting again we get:

${\displaystyle \left(1-{\frac {1}{3^{s}}}\right)\left(1-{\frac {1}{2^{s}}}\right)\zeta (s)=1+{\frac {1}{5^{s}}}+{\frac {1}{7^{s}}}+{\frac {1}{11^{s}}}+{\frac {1}{13^{s}}}+{\frac {1}{17^{s}}}+\ldots }$